Who would have thought that annoying hiss and the on screen ‘snow’ we experienced from time-to-time on our analog TVs was partly due to photons hanging around since the beginning of the universe interacting with the antenna and thereby providing evidence of the Big Bang? (The Big Bang channel as it has been called). Is was George Gamow, a Russian Cosmologist, who predicted that the Universe was filled with a residual radiation left over from the Big Bang, that had cooled to a few degrees above zero Kelvin. This Cosmic Background Radiation (CBR) was created 13.7 billion years ago which since then has been red-shifted to longer wavelengths due to the expansion of the Universe.
In the 1960s, Penzias and Wilson, two researchers for Bell Telephones, picked up this Big Bang relic for the first time using a microwave receiver. Today we can see this very uniform (with some irregularities or ripples) and very low energy radiation peaking in the microwave portion of the electromagnetic spectrum. It comes from all directions in space and has a characteristic temperature of just 2.7K. It is referred to as the ‘afterglow’ of that initial explosion and provides good supporting evidence for the Big Bang theory that says the universe began in a very hot and dense state but has expanded and cooled ever since. At the instant of the Big Bang spacetime was created along with all the matter and energy that exists today. This background radiation has been there since the dawn of time, and as the universe expanded, the radiation’s wavelength got longer, lost energy and got cooler, down to 2.7K that we measure it at today.
NASA’s Cosmic Background Explorer (COBE) satellite launched in 1989 was dedicated to exploring this background radiation and was able to make a historic discovery of tiny fluctuations, so-called ‘cosmic ripples’, in the temperature of the background radiation. These subtle temperature differences were vital because without them there would be no reason why matter would clump together. Instead it would be expected to be evenly distributed throughout the universe with no regions more dense or less dense than others. So there discovery was another strong piece of evidence supporting the Big Bang theory.
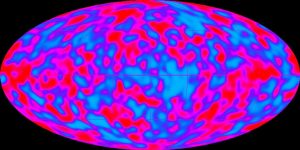
Of course it is also because of this almost precise uniform temperature that it causes a problem for the Big Bang model. This uniformity of the universe is a big problem. This is known as the horizon problem. We can look in one direction in space, right out to the edge of the known universe, some 46 billion light years, and measure the temperature of the CBR. Then we can look in the exact opposite direction again to the very edge and we find the same measured temperature. In fact, the measured temperature is the same regardless of the direction. This is a problem because the gap between both ends of the universe we have just measured the temperature of is 92 billion light years.
But the universe is only 14 billion years old. So how could both ends be at the same temperature if temperature equilibrium could only be reached if both ends of the universe were able to exchange information (heat radiation in this case), and this clearly cannot be the case as it would take 92 billion years to do so travelling at the speed of light. As we know, nothing can travel faster than the speed of light according to Einstein’s equations, so there is no way heat radiation could have traveled between the two horizons to even out the hot and cold spots created in the big bang and leave the thermal equilibrium we see now. Theories that may resolve this problem include the ‘inflation’ theory, and a theory that perhaps the speed of light was different in the early universe. If the inflation theory were true it would mean an ultra-fast (faster than light speed) expansion of the universe occurred soon after the Big Bang. But is this just a convenient theory? Why would inflation happen? Are we not just replacing one dilemma with another? And as of yet we have no evidence that the speed of light was different in the early universe either so we are still stuck on this ‘horizon problem’.
More later!